Home Site Map Search

GeoAstro Applets

Astronomy

Chaos Game

Java

Miscel- laneous

Jovian Moons Applet

The Galilean moons are the four moons of Jupiter discovered by Galileo Galilei in January 1610: Io, Europa, Ganymede and Callisto. They revolve around Jupiter with periods of 1.77 to 16.69 days, and have apparent magnitudes between 4.6 and 5.6 when Jupiter is in opposition with the Sun, and are about one unit of magnitude dimmer when Jupiter is in conjunction.

	Check your time zone offset and select the local standard time.
	Click the applet first! Enter latitude in decimal degrees, northern positive, southern negative, enter longitude in decimal degrees, eastern positive, western negative, then press the button "Apply input". 52.51° N, 13.41° E is Berlin, Germany.
	You may use the keys "m", "d", "h", "n" to increase the month, date, hour, minute, or Shift key and "m", "d", "h", "n" to decrease the month, date, hour, minute !
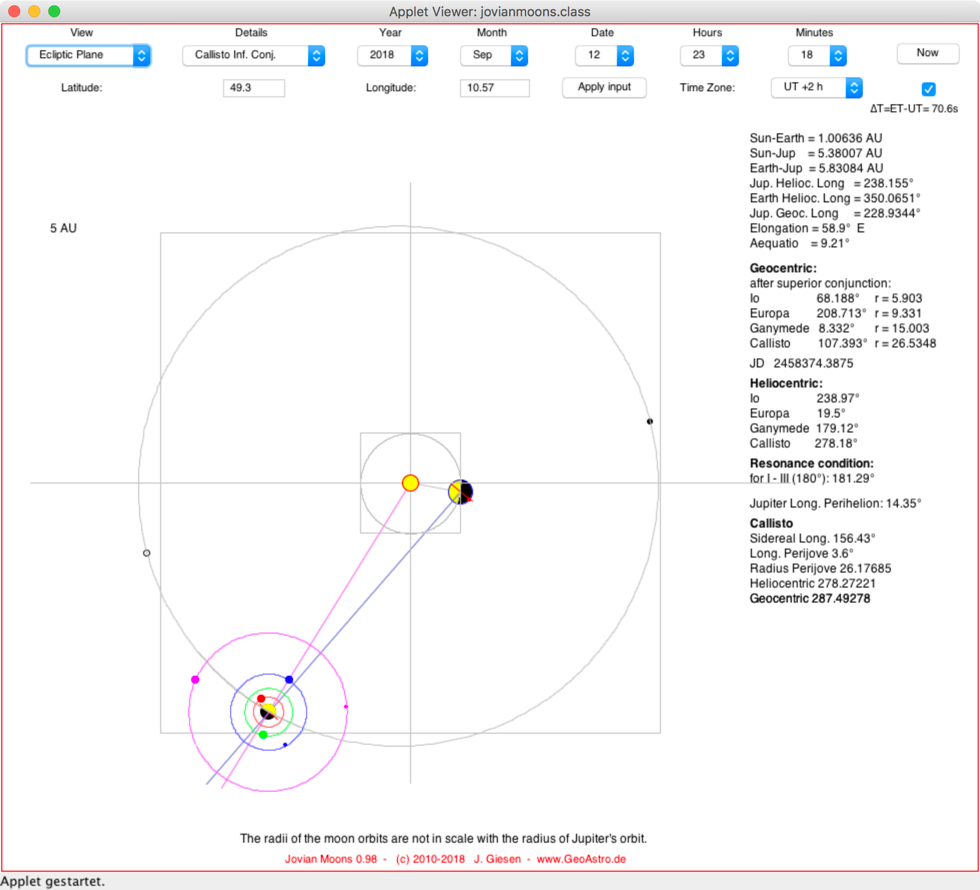
	The sizes of the moons are drawn according to their visual magnitudes (accuracy about +/- 0.1 mag). The angular distances from Jupiter, and the angle from inferior conjunction are at right.
	With "Orbit" selected from the "View" menu the illumination of Jupiter can be shown.
	The radii of Jupiter and the orbital radii of the satellites are drawn to scale.
	Blue: Jupiter visible in the local sky.
The simplified calculations used by the applet (according to Jean Meeus: Astronomical Algorithms) is neglecting the inclinations of the orbits of the satellites on the equatorial plane of Jupiter (0.04° for Io, 0.51° for Callisto): mutual occultations can not be calculated with certainty. For 1610 the accuracy of times of inferior conjunctions of Callisto was tested by comparison with CalSky, and the deviations were found to be less than 2 minutes.

Select "Diagram Moons" from the "View" menu:

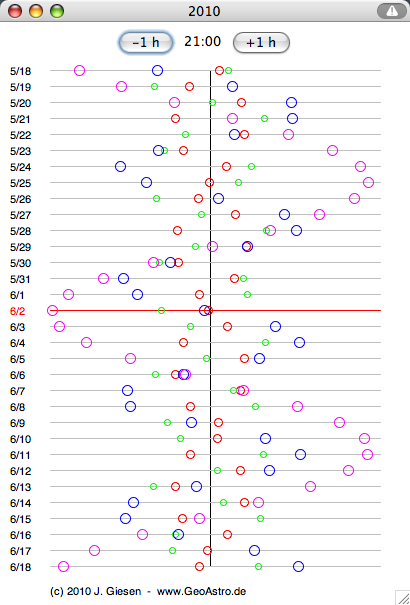
Select "Ecliptic Plane" from the "View" menu:

The three inner moons—Io, Europa, and Ganymede—are in a 4:2:1 orbital resonance with each other.
The Laplace resonance involving Io–Europa–Ganymede includes the following relation locking the orbital phase of the moons:

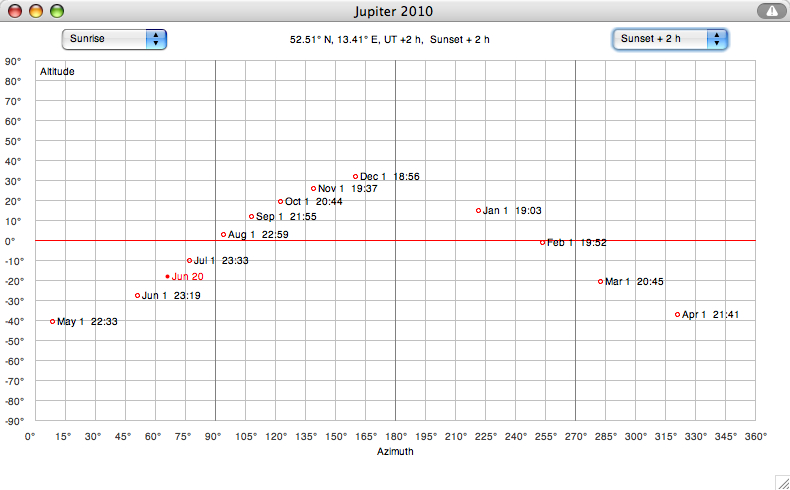
Select "Sky" from the "View" menu:
Jupiter's altitude and azimuth on the first of the month, 2 hours after sunset

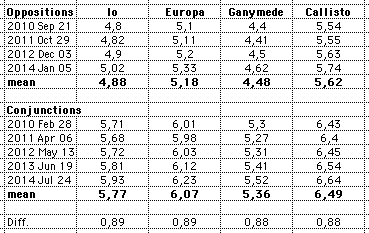
Extreme visual magnitudes of the Galilean Moons:

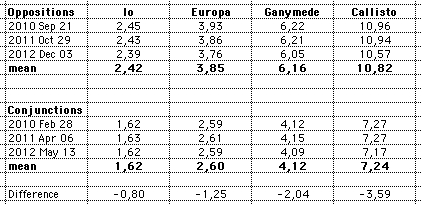
Extreme angular distances of the Galilean Moons from Jupiter (arc minutes):

Galilei's observations of 1610 January at Padova (45.4° N, 11.9° E) were published in
"Nuncius Sidereus" in Venice in March 1610.

The year 1610 can be selected from the "Year" menu:

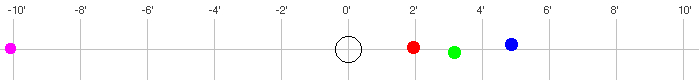
Jan 07:
Sunset 15:47 UT

  
Credit: Linda Hall Library of Science, Engineering and Technology, [Page 17 [sic].]*

Simulation at 17:00 UT:

Jan 08:
Callisto missing

          
Credit: Linda Hall Library of Science, Engineering and Technology, [Page 18 [sic].]*

Simulation at 17:00 UT:

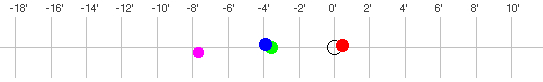
Jan 10:

Credit: Linda Hall Library of Science, Engineering and Technology, [Page 18 [sic].]* 

Simulation at 17:00 UT:

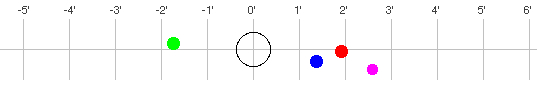
Jan 13:

Credit: Linda Hall Library of Science, Engineering and Technology

Simulation at 17:00 UT:

Sufficient separation for Galilei's perspicillum to observe four moons.

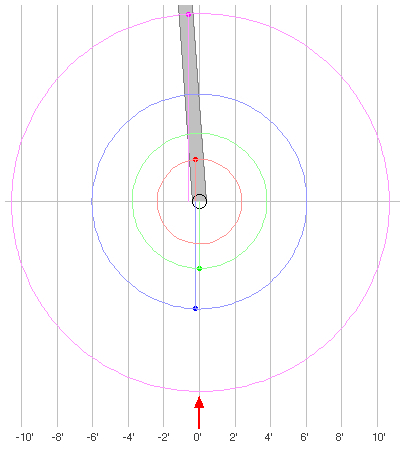
Jupiter seen without moons:

2009 Sep 3, 5:30 UT:
Io occulted/eclipsed,
Europa and Ganymede in transit,
Callisto in eclipse/transit.
Next event: 2019 Nov 9

Jupiter seen with one satellite only:

This event is occurs far more frequently.

2010 Mar 19 at 14:00 UT:

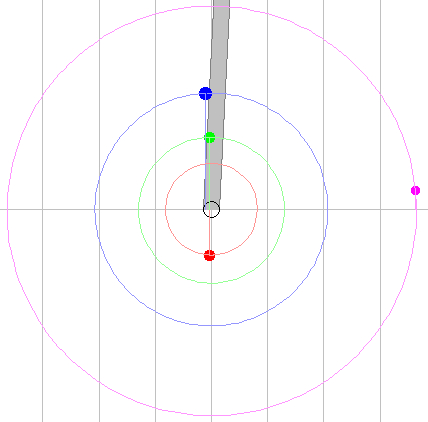
Io transit, Europa occulted/eclipsed, Ganymede occulted.

2010 Oct 27 at 15:00 UT:

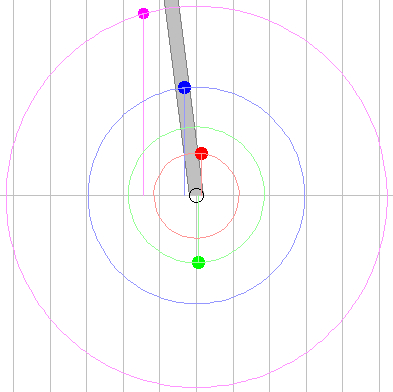
Io occulted, Europa transit, Ganymede eclipse.

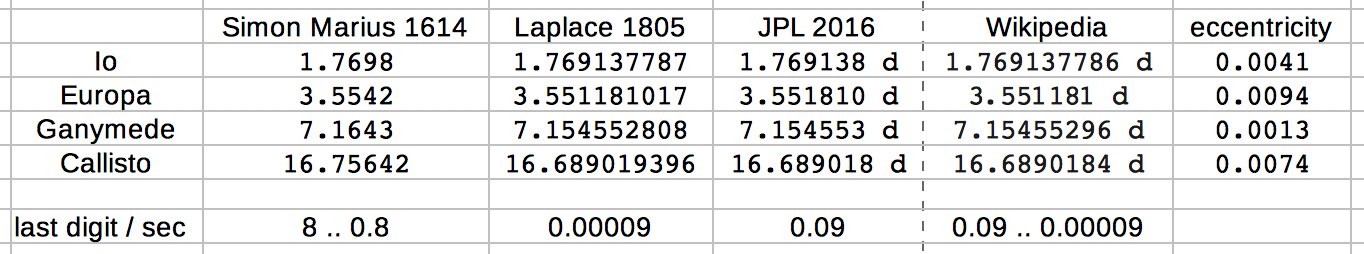
Orbital Periods as published by Simon Marius in 1614

Books

Simon Marius: Mundus Jovialis - Die Welt des Jupiter - Die Entdeckung der Jupitermonde durch den fränkischen Hofmathematiker und Astronimen Simon Marius im Jahr 1609 - lateinisch und deutsch. Herausgegeben und bearbeitet von Joachim Schlör, Schrenk-Verlag, Gunzenhausen, 1988. ISBN 3-924270-14-7 Wolfschmidt, Gudrun (Hg.): Simon Marius, der fränkische Galilei, und die Entwicklung des astronomischen Weltbildes. Tredition, Hamburg 2012. ISBN 978-3847238645 Galileo Galilei: Sidereus Nuncius - Nachricht von neuen Sternen. Hg. Hans Blumenberg, Suhrkamp 1980. ISBN 978-3518279373

Web Links

Simon Marius (Wikipedia) Simon Marius: Mundus Iovialis Anno M.DC.IX. Detectus Ope Perspicilli Belgici; Bayerische Staatsbibliothek digital The Mundus Jovialis of Simon Marius: Part 1, Part 2, Part 3, Part 4 Galilean moons (Wikipedia) Jovian Satellite Fact Sheet (NASA) Jupiter (Wikipedia) Jovian system simulator (high accuracy) Jupiter's Moon (simulation, Sky & Telescope) Javascript Jupiter Galilean Moons Applet Phenomena of the Galilean Satellites of Jupiter (IMCE) Galilei: Nuncius Sidereus (Linda Hall Library of Science, Engineering and Technology) From Occhiale to Printed Page: The Making of Galilei's Nuncius Sidereus (O. Gingerich, A. van Helden, PDF) Jupiter satellite events and GRS times for 2012-2013 Orbital resonance (Wikipedia) Sinclair, A. T.: The Orbital Resonance amongst the Galilean Satellites of Jupiter, Mon. Not. R. astr. Soc. (175), 171, 59-72. Paita, F. et al.: Element history of the Laplace resonance: a dynamical approach Laplace, P.-S.: Traite de Mecanique Celeste Vol. IV, Paris 1805, chez Courcier. page 16 HORIZONS Web-Interface (JPL) De Sitter, W.: Orbital Elements Determining the Longitudes of Jupiter’s Satellites derived from Observations. Annalen van de Sterrrewachtte Leiden, Deel XVI, tweede stuck, 1928. WolframAlpha Widgets Regression Calculator

Last Modified: 2023, Oct 05

© 2010-2023 Juergen Giesen